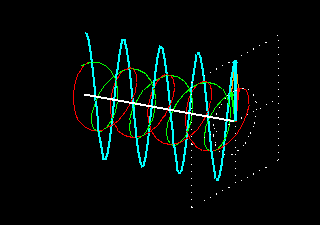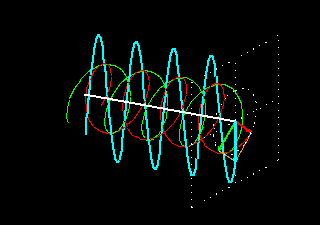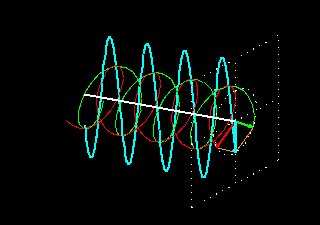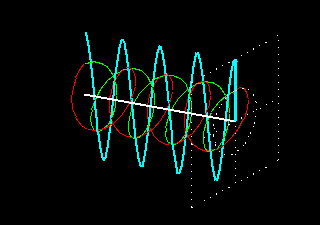
As we have seen in the previous pages, two plane-polarized waves can meet. Of course, two circularly polarized waves can meet as well. In that case, the fields are added according to the rules of vector addition, just as with plane-polarized waves.
The superposition of two circularly polarized light beams can result in various outcomes. The most interesting result is obtained when a left circularly polarized wave and a right circularly polarized wave are added. Let's assume that the two light beams have equal amplitudes and wavelengths. The following animation shows this superposition and the resulting wave (red and green colors indicate the two superposing components and light blue indicates the resulting wave):

When the intersecting plane is looked at from the front then the following image is seen:
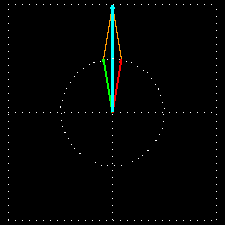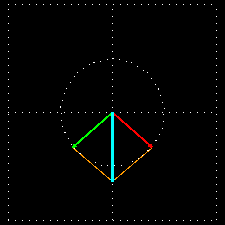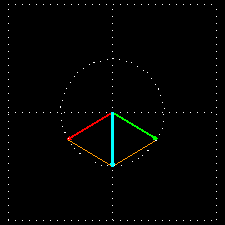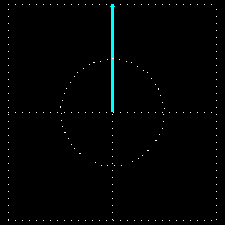
(Attention! The two animations are not synchronized!)
As we see, the result of superposing two circularly polarized waves is a plane-polarized wave.
By thinking "backwards", we can reach the following very important conclusion: Any linearly polarized light wave can be obtained as a superposition of a left circularly polarized and a right circularly polarized light wave, whose amplitude is identical.